The Decay Risk of Investing in Multi-fold Leveraged ETFs

An ETF, or Exchange Traded Fund, is an investment tool that, similar to stocks, can be bought and sold on stock exchanges. However, unlike individual stocks, an ETF usually comprises a basket of assets such as stocks, bonds, or commodities. By purchasing shares of an ETF, investors can indirectly own these assets and earn investment returns. Like stocks, ETFs are traded on securities exchanges.

Leveraged ETFs, often referred to as "intraday leveraged ETFs," use tools such as borrowing or derivatives to increase the leverage of an investment portfolio, aiming to achieve higher returns in the market. Examples include the triple-leveraged inverse Nasdaq ETF ProShares Ultra QQQ (SQQQ) and the triple-leveraged Nasdaq ETF ProShares UltraPro QQQ (TQQQ). Both track the Nasdaq index, but they achieve a triple effect by additionally purchasing index futures and other derivative products. Common leverage ratios include 1.5X, 2X, and 3X, and there are typically pairs of leveraged ETFs that take long and short positions.

While multiple leverage can amplify investment returns, leveraged ETFs have higher risks compared to regular ETFs, with leverage decay being one of the risks involved.

Leveraged decay refers to the process by which leveraged ETFs strictly adhere to a "daily rebalancing" rule to ensure that they consistently achieve an N-times tracking effect by the end of the day or before the next trading day opens, resulting in decay.

Let's illustrate how a triple-leveraged ETF can cause decay in its net asset value with a simple example:

1)The initial net asset value of the leveraged ETF is $10,000. Using triple leverage, the amount borrowed is $20,000, resulting in a total asset value of $30,000.

2)If the index rises by 1% on a given day, the corresponding triple-leveraged ETF should rise by 3% (three times). The total assets increase to: $30,000 × (1 + 1%) = $30,000 × 1.01 = $30,300. Subtracting the borrowed amount of $20,000, the final net asset value is: $30,300 - $20,000 = $10,300.

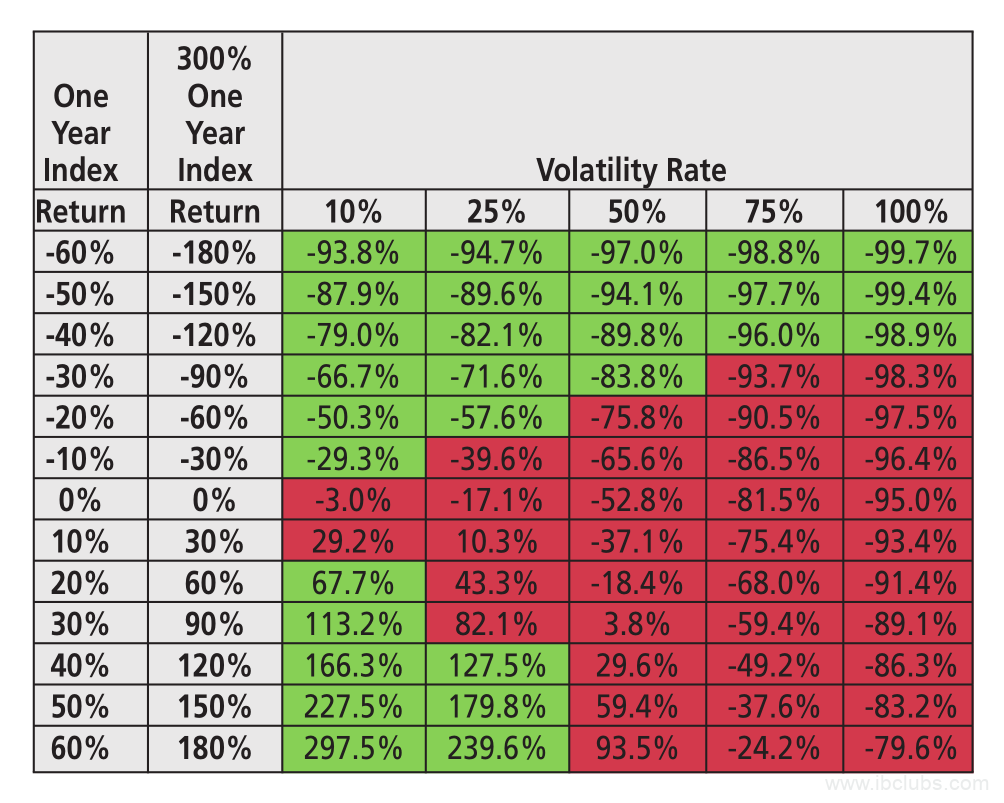
3)Before the close of the day, to ensure that the tracking effect remains at 3 times the following day, it is necessary to borrow three times the new net asset value of $10,300, resulting in total assets of $10,300 × 3 = $30,900. The additional amount borrowed is: ($30,900 - $10,300) - $20,000 = $600, making the total borrowed amount $20,600.

4)Assuming that on the second trading day, the index falls by 1%, the corresponding triple-leveraged ETF would fall by 3% (three times). The total assets decrease to: $30,900 × (1 - 1%) = $30,900 × 0.99 = $30,591. Subtracting the total borrowed amount of $20,600, the net asset value of the leveraged ETF is: $30,591 - $20,600 = $9,991, resulting in decay.

This decay occurs because leveraged ETFs involve "leveraged borrowing to buy" and "daily position adjustments." When the market rises on a given day, the net asset value of the leveraged ETF also increases. To ensure the tracking effect for the next day, it is necessary to "increase borrowing/risk exposure" before the close of trading (because the net value of the leveraged ETF has increased). If the market falls the next day, due to the increased total market value, the loss in net asset value caused by the fall will be greater.

There are many factors that can affect the returns of leveraged ETFs, including the volatility of the index, the return rate of the index, the length of time the leveraged ETF is held, the size of the leverage ratio in the ETF, and whether dividends/interest are received, among others. The two biggest influencing factors are the "index return rate" and "index volatility." If the index fluctuates within a year without a clear upward or downward trend, investors will continuously suffer from leverage decay, as well as transaction costs such as borrowing interest and high management fees. As a result, it is highly probable that holding a leveraged ETF will lead to a loss. Even if the index rises, if the volatility within the year is too high, holding a leveraged ETF can still result in a loss.

The following hypothetical scenario illustrates the impact of "index return rate" and "index volatility" on leveraged ETFs (assuming no dividends, no ETF fees, and a 0% borrowing rate):

From the graph, we can see that assuming the index has an annualized return of 0, if the index's volatility is 10%, then the leveraged ETF loses 3%. If the index's volatility is 25%, then the leveraged ETF loses 17.1%. Moreover, if the index rises by 10%, and the index's volatility for the year exceeds 50%, then the leveraged ETF would still incur a loss of more than 37%.

Therefore, due to the characteristics of leveraged ETFs, they are not recommended for long-term holding. These ETFs are primarily intended for short-term trading, aiming to achieve excess returns beyond the index's movements up or down. In a volatile market, the decay of leveraged ETFs becomes more pronounced. Once the cumulative decay is too high and the tracking error of the index becomes significant, it would require a greater rise to recover the losses.

If one is truly considering a long-term hold of a leveraged ETF, it would only be suitable in a market with a consistent upward or downward trend, as the returns would have to justify the risks faced.